Unit 8 right triangles & trigonometry – Embark on a captivating journey into Unit 8: Right Triangles and Trigonometry, where the interplay of geometry and measurement unveils a realm of intriguing concepts and practical applications. Prepare to delve into the intricacies of right triangles, unravel the mysteries of trigonometric ratios, and witness the transformative power of trigonometric functions.

As we navigate this mathematical landscape, we will explore the fundamental properties of right triangles, unlocking the secrets of the Pythagorean Theorem and its profound implications. We will delve into the world of trigonometric ratios, understanding how they quantify the relationships between sides and angles, and empowering us to solve complex problems.

Right Triangle Properties

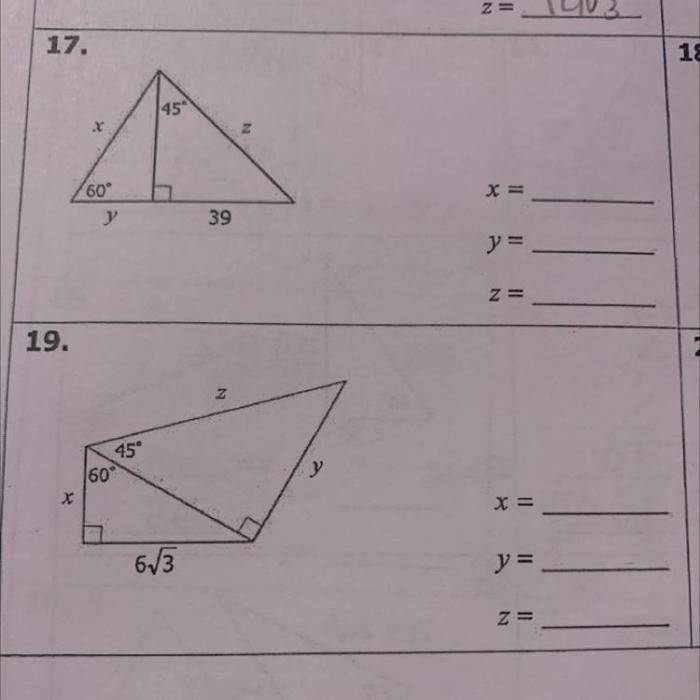
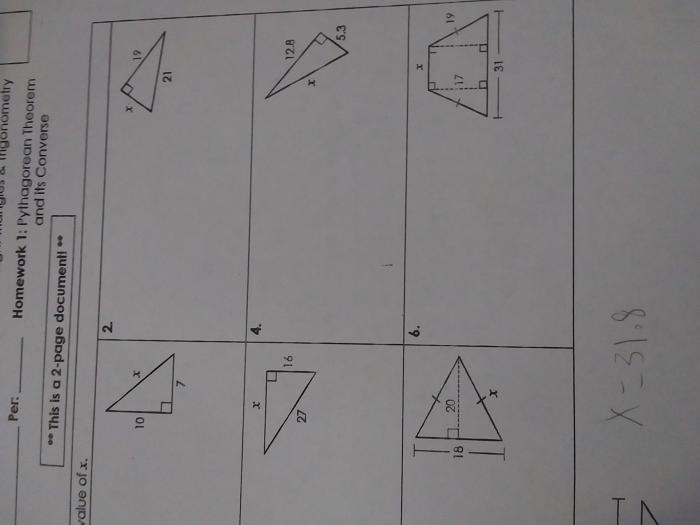
Right triangles are triangles with one right angle (90 degrees). The Pythagorean Theorem is a fundamental property of right triangles that states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.

Pythagorean Theorem

• Formula: a²+ b²= c², where aand bare the lengths of the two shorter sides (legs) and cis the length of the hypotenuse.
• Applications:
  • Finding the length of a missing side of a right triangle.
  • Determining the distance between two points in a coordinate plane.
  • Calculating the height of a building or tree.

Other Properties of Right Triangles, Unit 8 right triangles & trigonometry

• Angle-Angle-Side (AAS) Relationship:If two angles and one side of a triangle are known, then the triangle is uniquely determined.
• Side-Angle-Side (SAS) Relationship:If two sides and one angle of a triangle are known, then the triangle is uniquely determined.

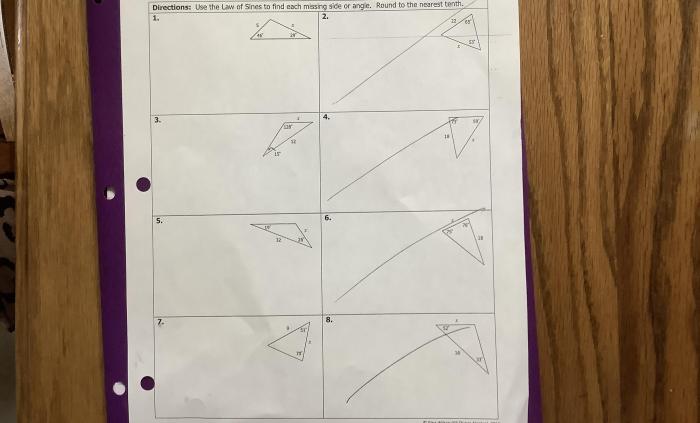
Trigonometric Ratios

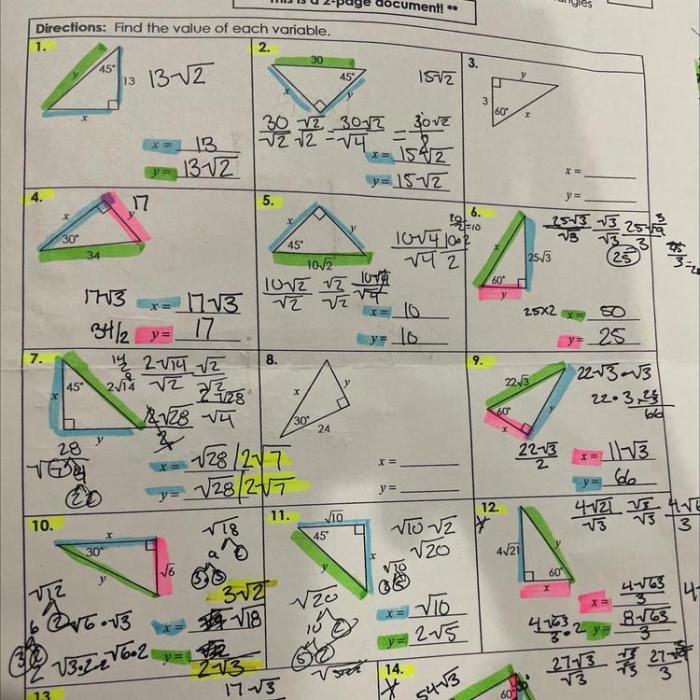
Trigonometric ratios are functions that relate the lengths of the sides of a right triangle to the angles of the triangle. The three main trigonometric ratios are sine, cosine, and tangent.

Definition of Trigonometric Ratios

• Sine (sin):The ratio of the length of the opposite side to the length of the hypotenuse.
• Cosine (cos):The ratio of the length of the adjacent side to the length of the hypotenuse.
• Tangent (tan):The ratio of the length of the opposite side to the length of the adjacent side.

Calculating Trigonometric Ratios

Trigonometric ratios can be calculated using the Pythagorean Theorem and the definitions of the ratios.

Applications of Trigonometric Ratios

• Finding the height or distance of an object.
• Solving problems involving angles of elevation or depression.
• Determining the direction of a moving object.

Trigonometric Functions

Trigonometric functions are mathematical functions that represent the trigonometric ratios of angles. The three main trigonometric functions are sine, cosine, and tangent.

Definition of Trigonometric Functions

• Sine function (sin x): The function that gives the sine of an angle x.
• Cosine function (cos x): The function that gives the cosine of an angle x.
• Tangent function (tan x): The function that gives the tangent of an angle x.

Graphing Trigonometric Functions

Trigonometric functions can be graphed by plotting the values of the function for different values of the angle.

Properties of Trigonometric Functions

• Periodicity:Trigonometric functions are periodic functions with a period of 2π.
• Symmetry:Sine and cosine functions are even functions, while tangent function is an odd function.

Applications of Trigonometry: Unit 8 Right Triangles & Trigonometry

Trigonometry is used in a wide variety of real-world applications, including navigation, surveying, and architecture.

Navigation

• Trigonometry is used to calculate the distance and direction between two points on a map.
• It is also used to determine the course and speed of a ship or aircraft.

Surveying

• Trigonometry is used to measure the height of buildings and trees.
• It is also used to determine the distance between two points on the ground.

Architecture

• Trigonometry is used to design and build structures such as bridges and buildings.
• It is also used to calculate the angles of roofs and other architectural features.

FAQ Corner

What is the Pythagorean Theorem?

The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.

What are trigonometric ratios?

Trigonometric ratios are functions that relate the lengths of the sides of a right triangle to the angles between them. The three main trigonometric ratios are sine, cosine, and tangent.

What are trigonometric functions?

Trigonometric functions are mathematical functions that represent the trigonometric ratios of angles. They are used to model periodic phenomena, such as the motion of a pendulum or the rotation of the Earth.